Dark Star
Awards
Northeastern NM Regional Science and Engineering Fair: 1st Place in Junior Physics Category.
Northeastern New Mexico Junior Academy of Science Scientific Research Paper Junior Competition: 2nd Place.
New Mexico Science and Engineering Fair: 1st Place in Junior Physics Category.
Research Question
How will interstellar bodies of different masses entering the Solar System affect the probability for life on Earth to go extinct?
Hyphothesis
If an interstellar object enters the Solar System, then the Earth's orbit will be affected the most by the bodies of at least one solar mass because those are as great as that of the sun, so they will affect the Earth the most.
Introduction
Theory
Types of Stars
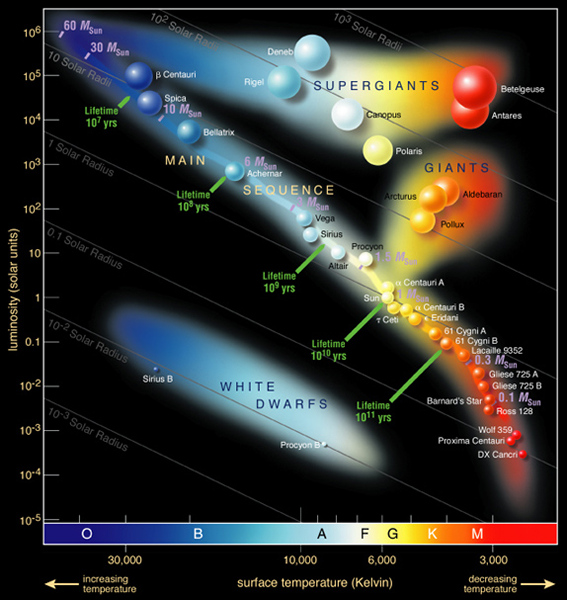
Figure 1: Hertzsprung-Russell (H-R) diagram[1]
The Hertzsprung-Russell (H-R) diagram is a star chart that classifies stars by temperature and luminosity.
It defines different types of stars:
- Main sequence stars
- Giants
- White dwarfs
- Brown dwarfs
The stars that are used in this project are:
- Brown dwarf - 0.01 to 0.05 Solar masses ()
- Black dwarf - 1 Solar mass
- Stellar black hole - 5 Solar masses
Solar System
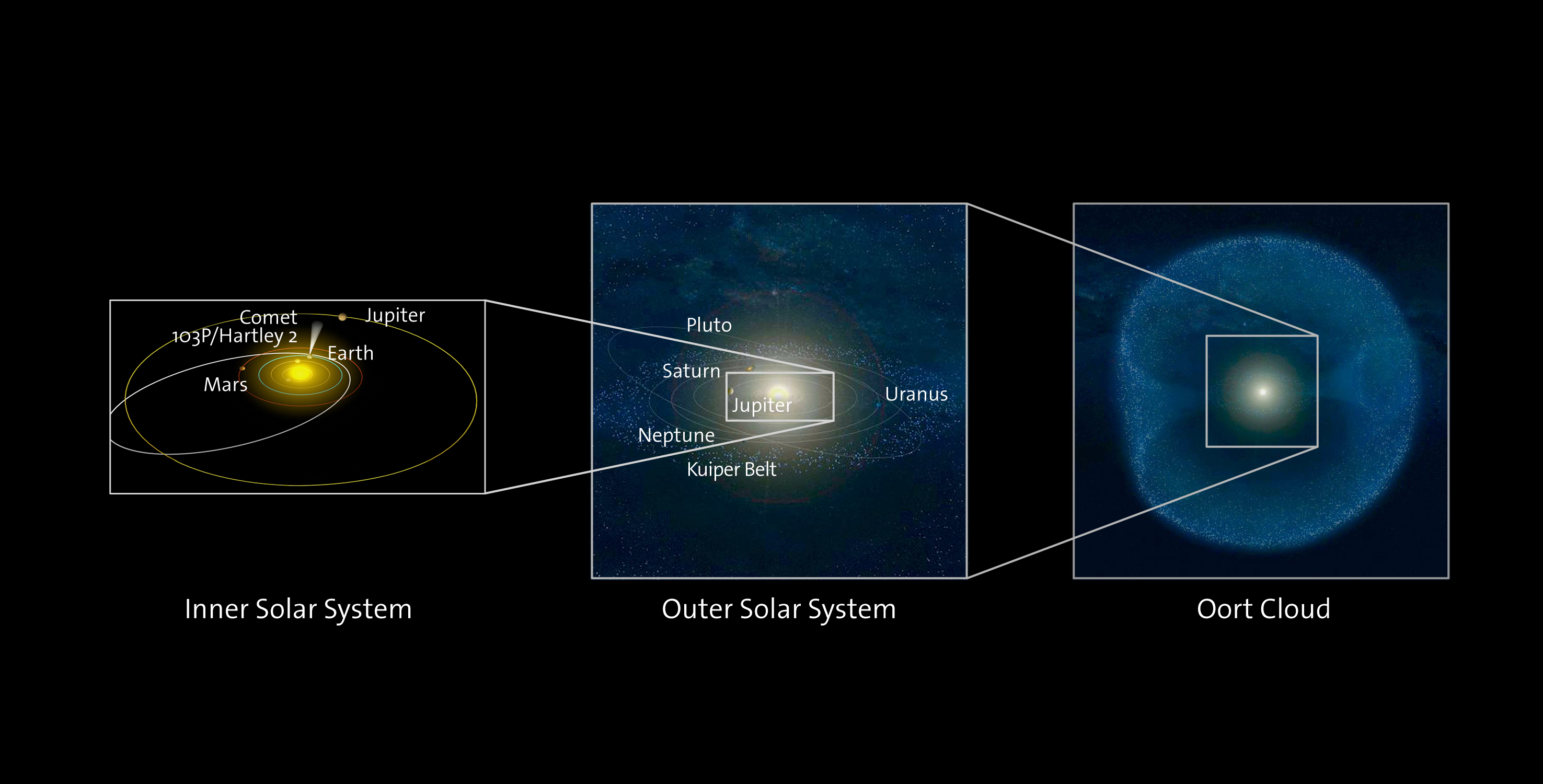 Figure 2: The Solar System[2]
Figure 2: The Solar System[2]
Solar System Regions
Inner planets
- Rocky, warm, small planets
- "Habitable Zone" - zone in which life can survive (0.8 AU - 2 AU from the Sun).
Asteroid belt: area of asteroids that separates the inner from the outer planets
Outer planets: gaseous, cold, massive planets
Kuiper belt: belt of asteroids, comets, and dwarf planets past the orbit of Neptune
Heliosheath: region of space in which solar wind is stopped by interstellar wind
Oort cloud: hypothetical region of space thought to contain the long term comets that orbit the Sun
Velocities
| Body | AU/day |
|---|---|
| Mercury | 0.028 |
| Venus | 0.02 |
| Earth | 0.017 |
| Mars | 0.014 |
| Jupiter | 0.0075 |
| Saturn | 0.0056 |
| Uranus | 0.0039 |
| Neptune | 0.0031 |
| Oumuamua | 0.015 |
| Luhman 16 | 0.012 |
| Proxima Centauri | 0.013 |
| Barnard's Star | 0.063 |
Interstellar Neighborhood
Single Stars
- Barnard's Star - 5.9 light years ()
- WISE 0855-0714 - 7.3 light years ()
- Wolf 359 - 7.8 light years ()
- Lalande 21185 - 8.3 light years ()
Binary Systems
Alpha Centauri - 4.3 light years
- Alpha Centauri A ()
- Alpha Centauri B ()
Luhman 16 - 6.5 light years
- Luhman A ()
- Luhman B ()
Sirius - 8.6 light years
- Sirius A ()
- Sirius B ()
Earth's life can be destroyed in several ways. One way is if asteroids or comets collide with Earth. This can be caused by the interstellar object disrupting the Oort cloud or the Kuiper or asteroid belt.
Another reason for life's extinction can be if the gravitational force of the interstellar body (or one of the planets) knocks off the Earth out of the Habitable Zone. This event will be the focus for my project.
N-body Simulator: Rebound
https://rebound.readthedocs.io/en/latest/
- N-body simulator
- Python and C extensible
- Good documentation and examples
- Can show the planets and stars trajectories while the simulation is running
Approach
- Create an N-body gravitational simulator.
- Add the bodies of the solar system to the simulation.
- Add the different dark stars to the program.
- For each simulation, change the position and the trajectory of the dark star.
- After using all the velocities assigned to one dark star, change the mass.
- Repeat Steps 4 and 5 for the all three types of dark bodies.
- Find probability of Earth leaving the Habitable Zone, maximum distance that the star can get to the Sun while affecting Earth, and the minimum distance that the star can get to the Sun that does not affect Earth.
Testing Parameters
- Simulated time: 200 years
Dark star masses (in ):
- Brown dwarf - 0.05
- Black dwarf - 1
- Stellar black hole - 5
Spherical coordinate system:
- Uses , instead of , , and .
Varying and form a sphere around the center.
Starting position:
- Distance = 50 AU
- and change by 8 degrees.
Starting trajectory:
- Velocities (AU/day): 0.001, 0.003, 0.005, 0.01, 0.015, 0.02, 0.025, 0.06
- Direction: and change by 7 degrees.
Simulations:
- Approximately 700,000 simulations for each velocity on each mass.
- Total of 16,800,000 simulations.
Results
Probability of Earth's Life Extinction
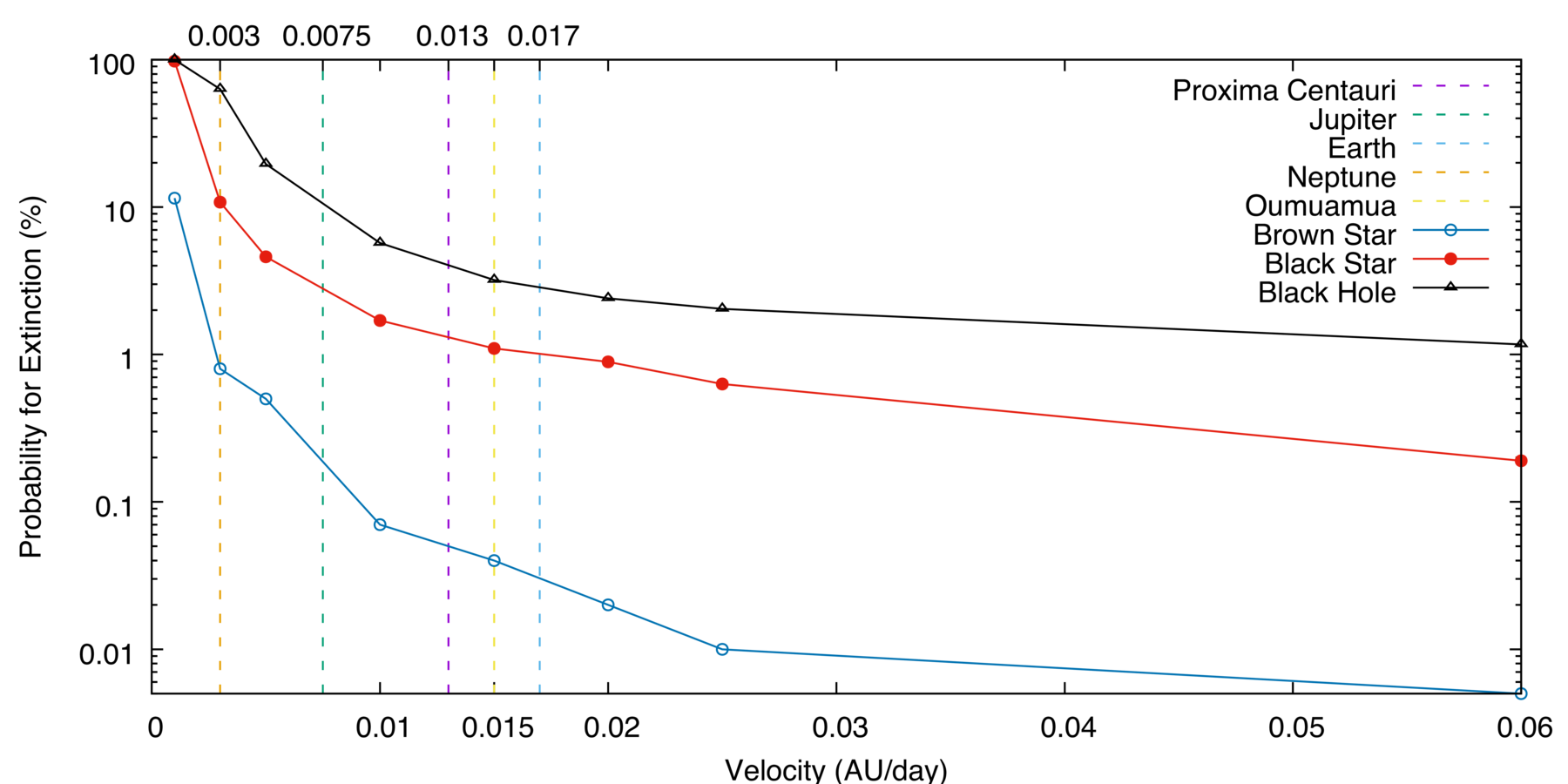 Figure 3: Effect of velocity on probability of Earth's life extinction
for simulated dark stars
Figure 3: Effect of velocity on probability of Earth's life extinction
for simulated dark stars
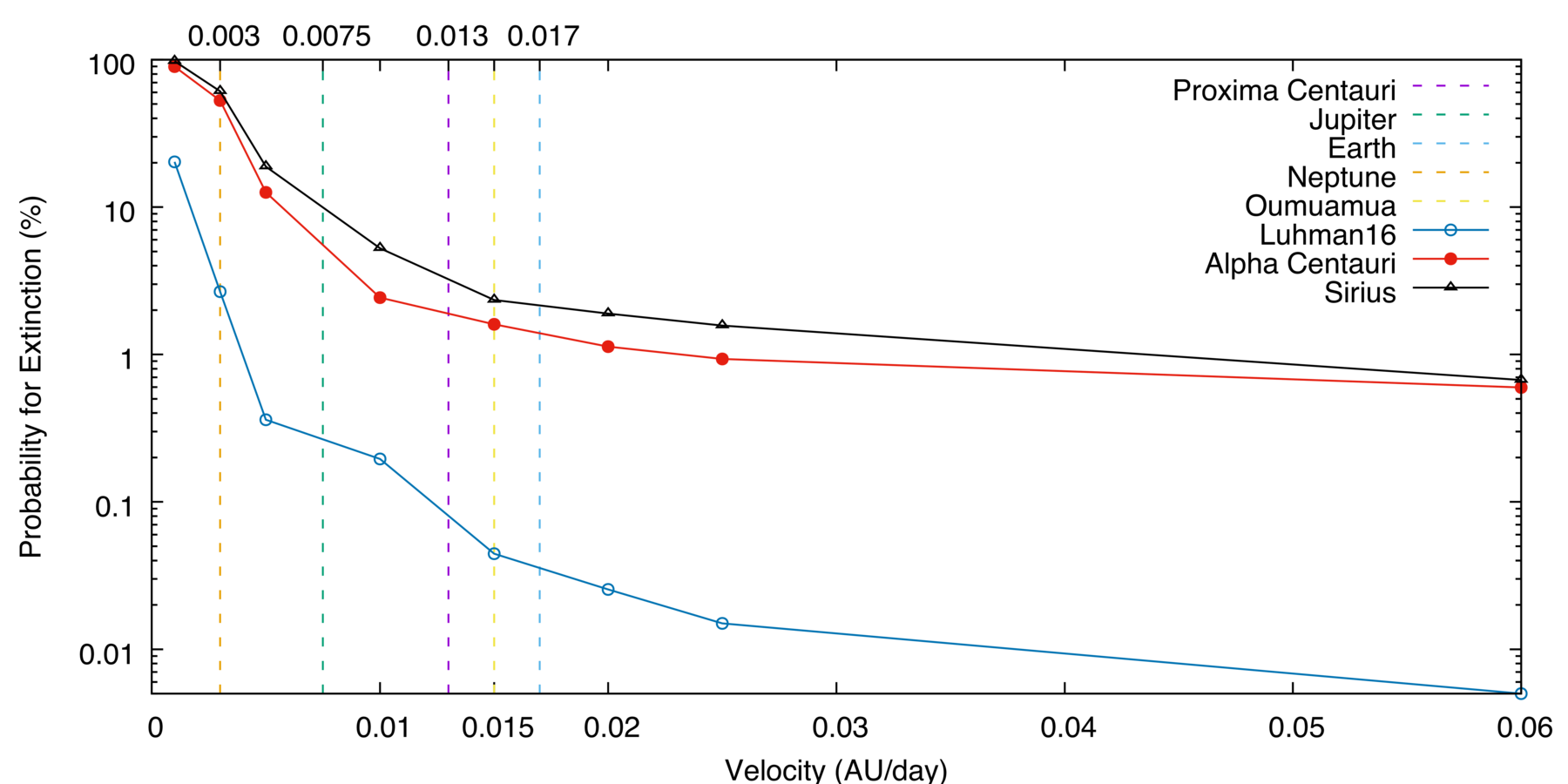 Figure 4: Effect of velocity on probability of Earth's life extinction
for three neighboring binary systems
Figure 4: Effect of velocity on probability of Earth's life extinction
for three neighboring binary systems
Other Results
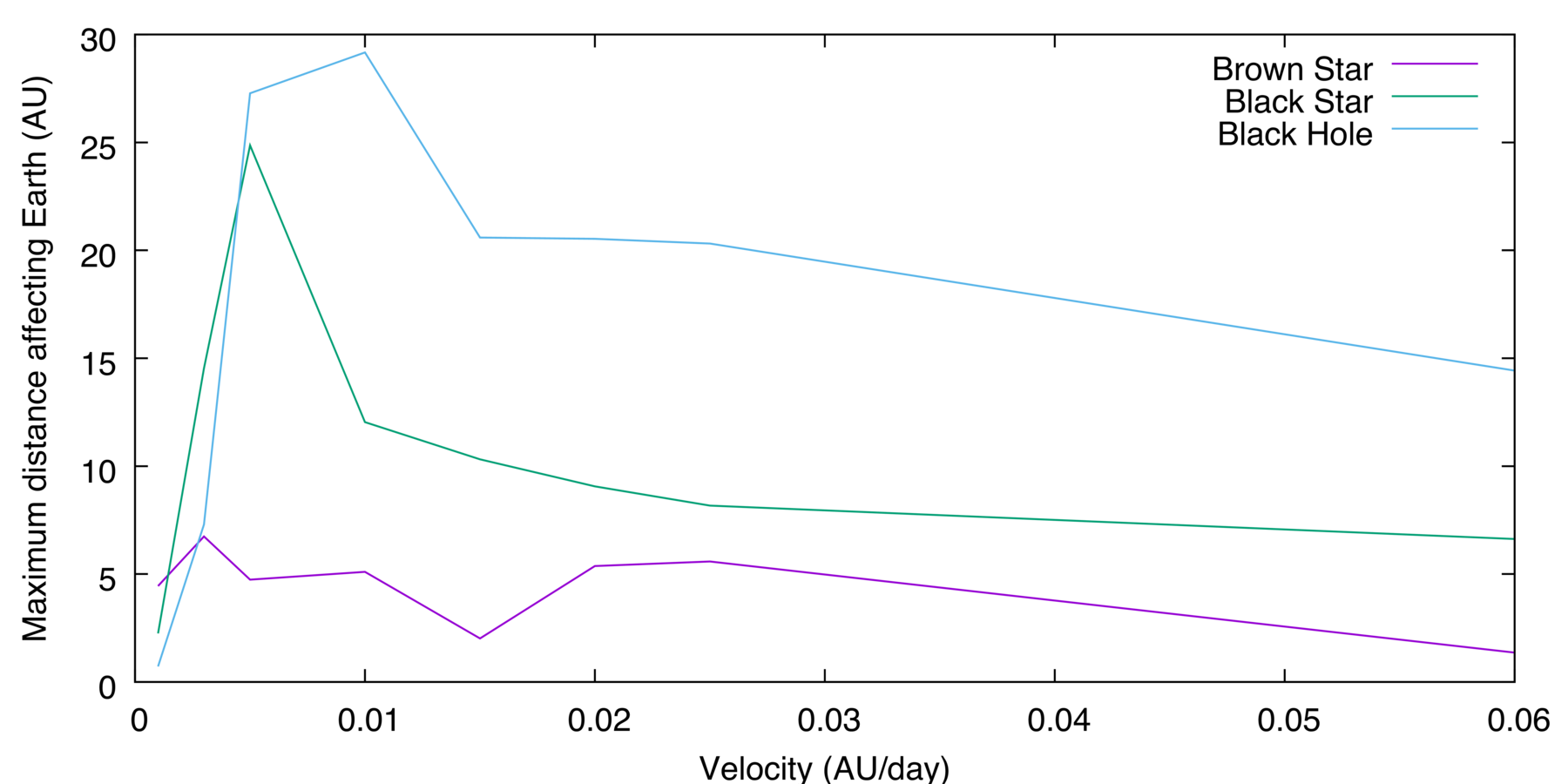 Figure 5: Maximum distance from the Sun that still affects the Earth
for simulated dark stars
Figure 5: Maximum distance from the Sun that still affects the Earth
for simulated dark stars
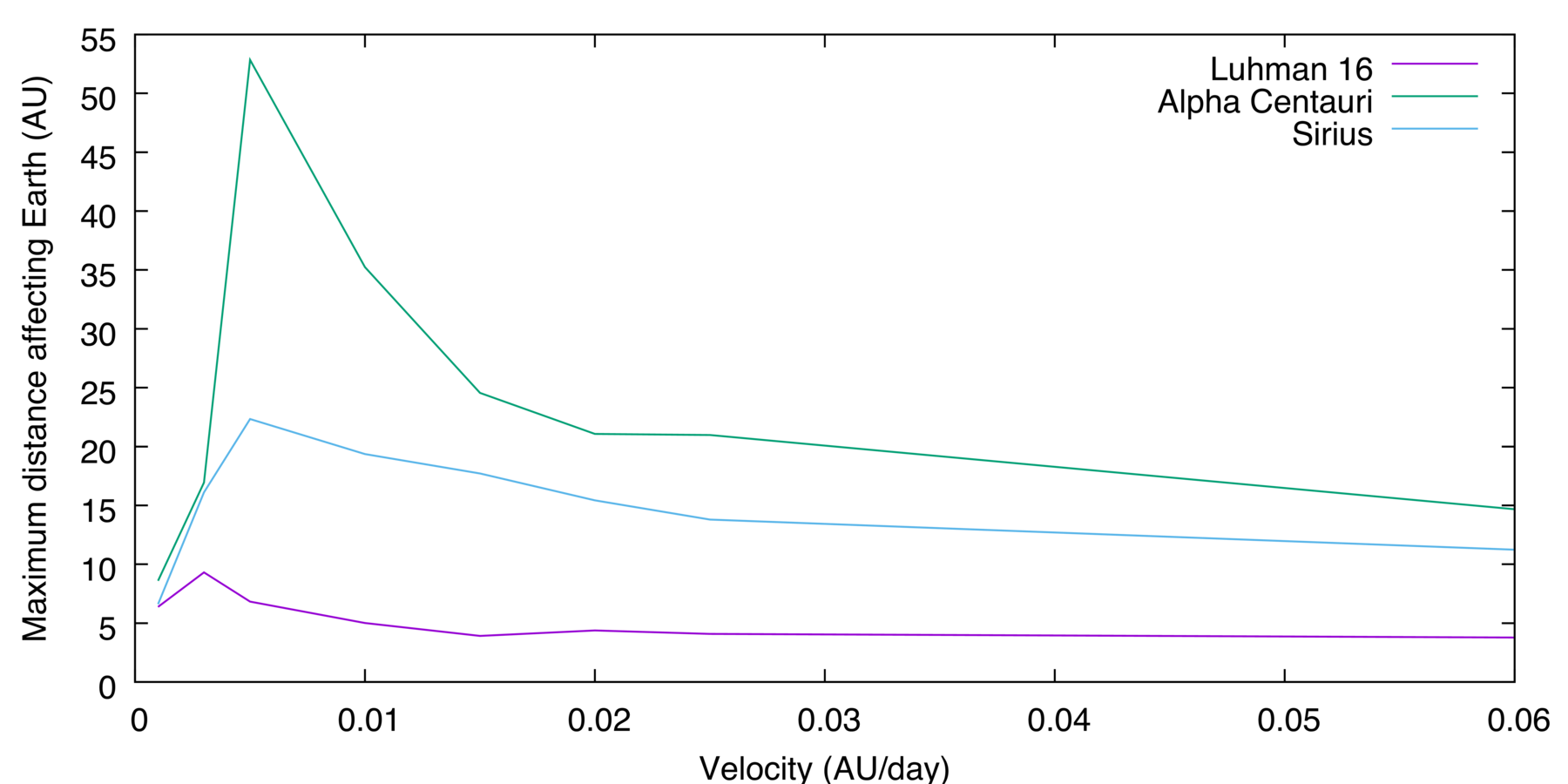 Figure 6: Maximum distance from the Sun that still affects the Earth
for binary systems
Figure 6: Maximum distance from the Sun that still affects the Earth
for binary systems
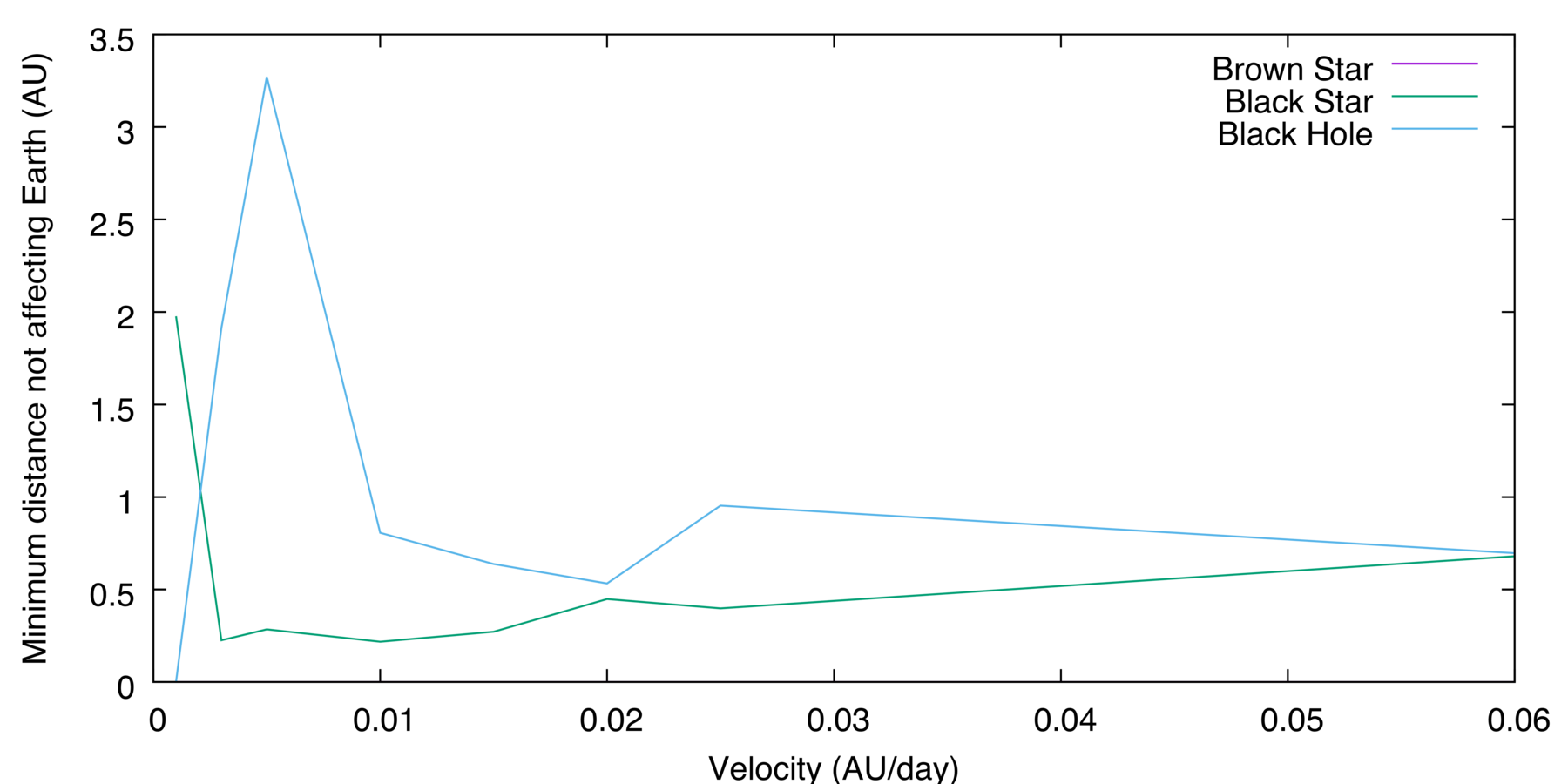 Figure 7: Minimum distance from the Sun that does not affect Earth for
simulated dark star
Figure 7: Minimum distance from the Sun that does not affect Earth for
simulated dark star
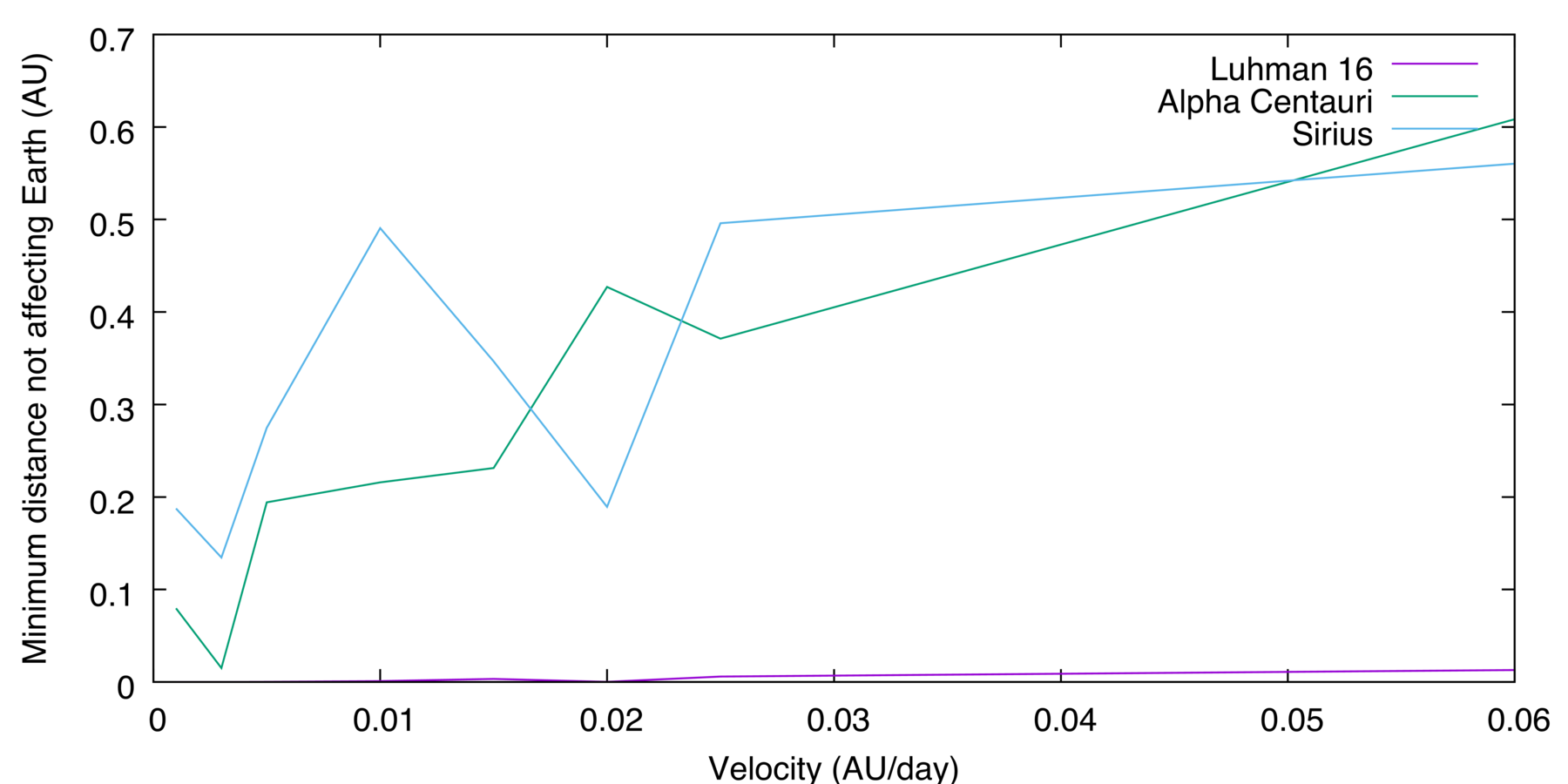 Figure 8: Minimum distance from the Sun that does not affect Earth for
binary systems
Figure 8: Minimum distance from the Sun that does not affect Earth for
binary systems
Brown Dwarf: Effects on Earth's Orbit
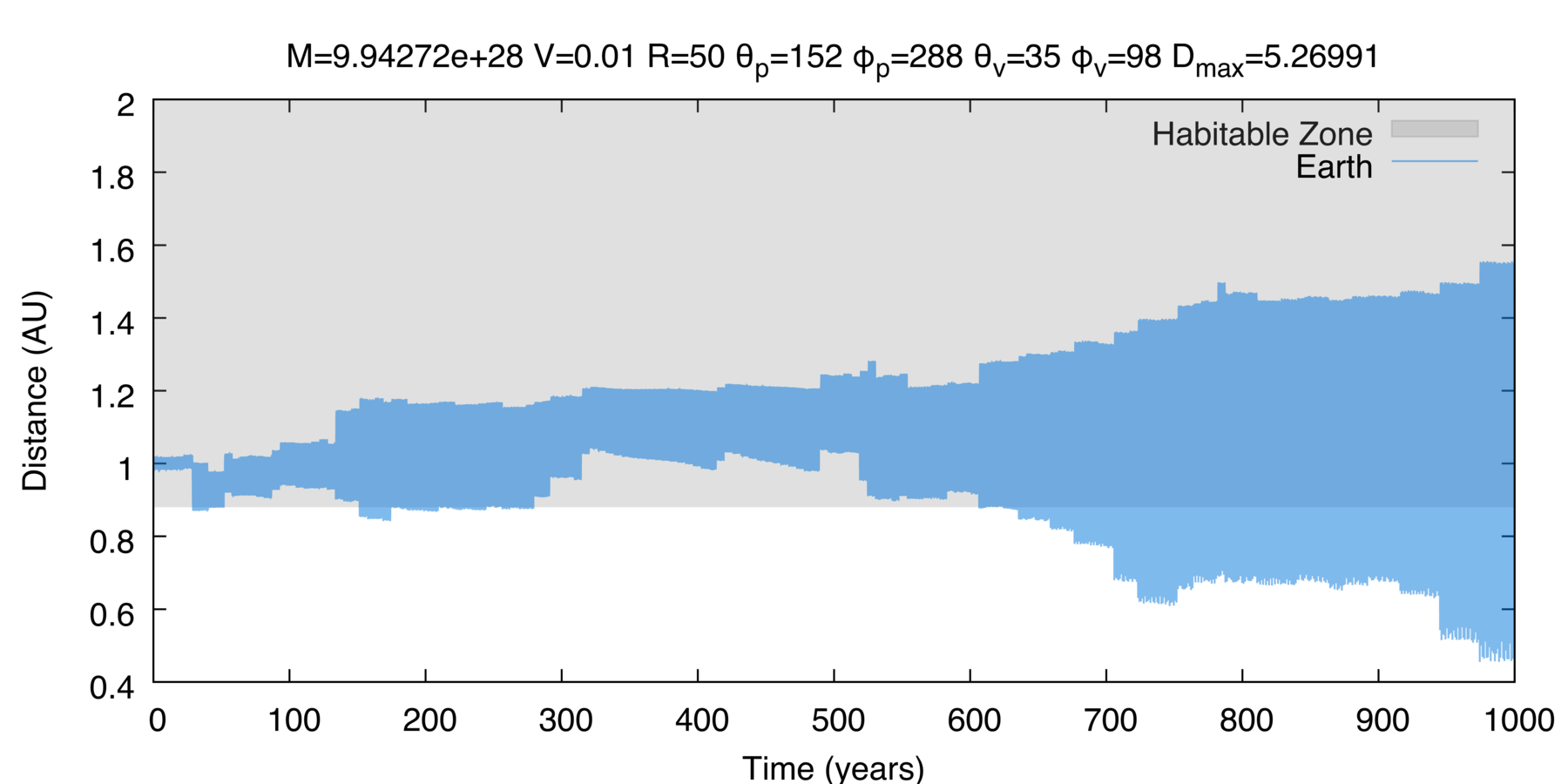
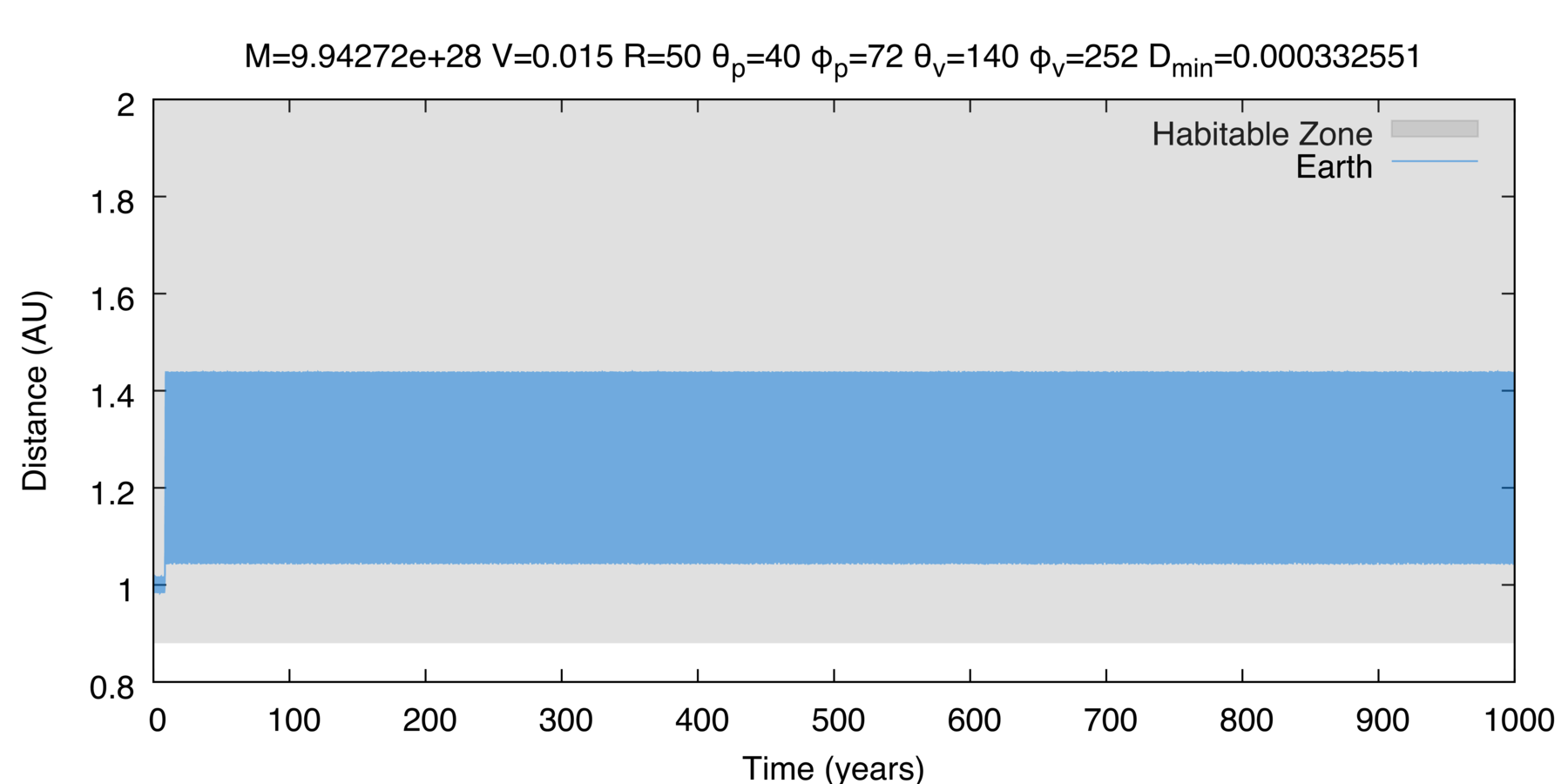
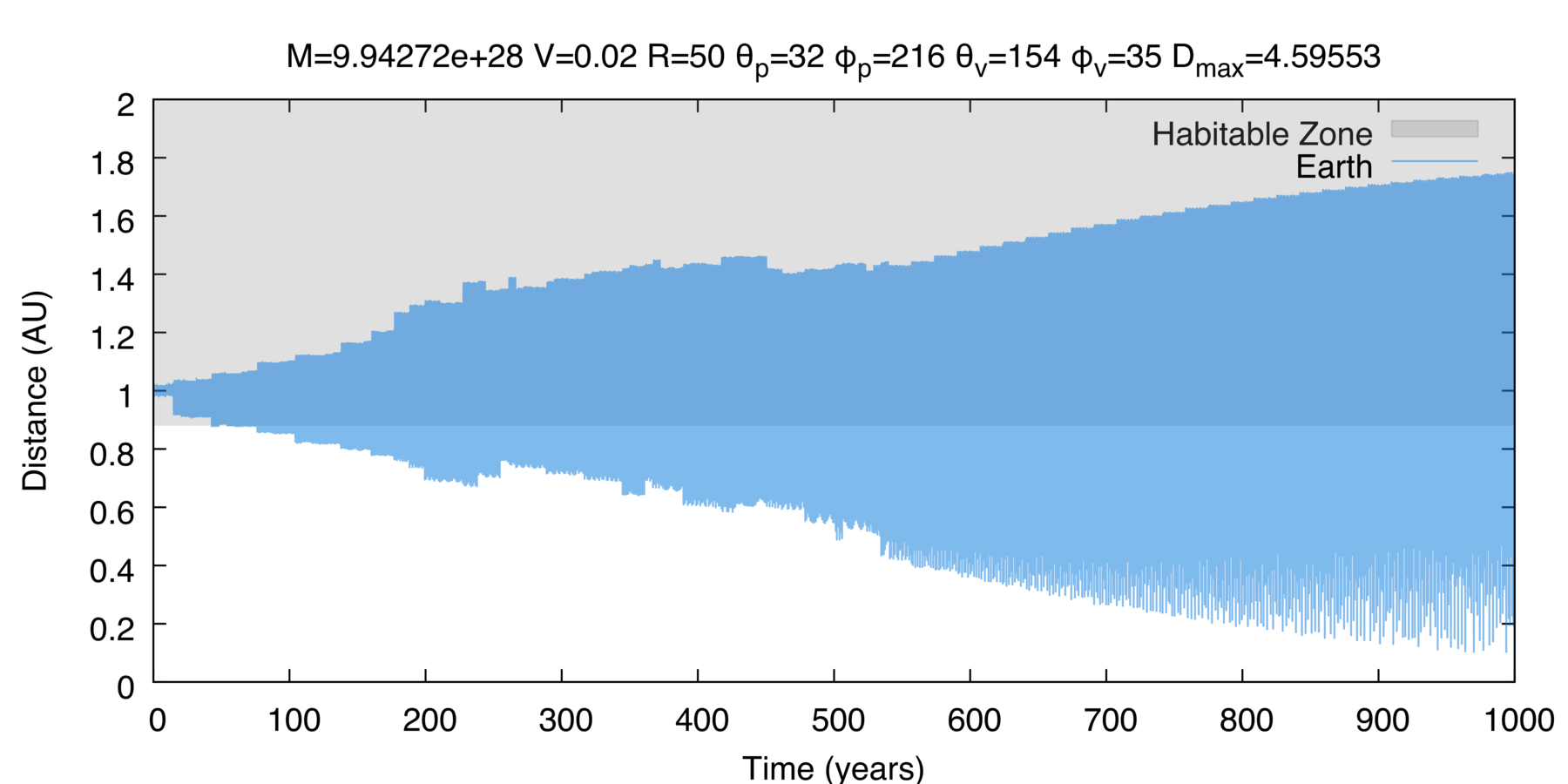
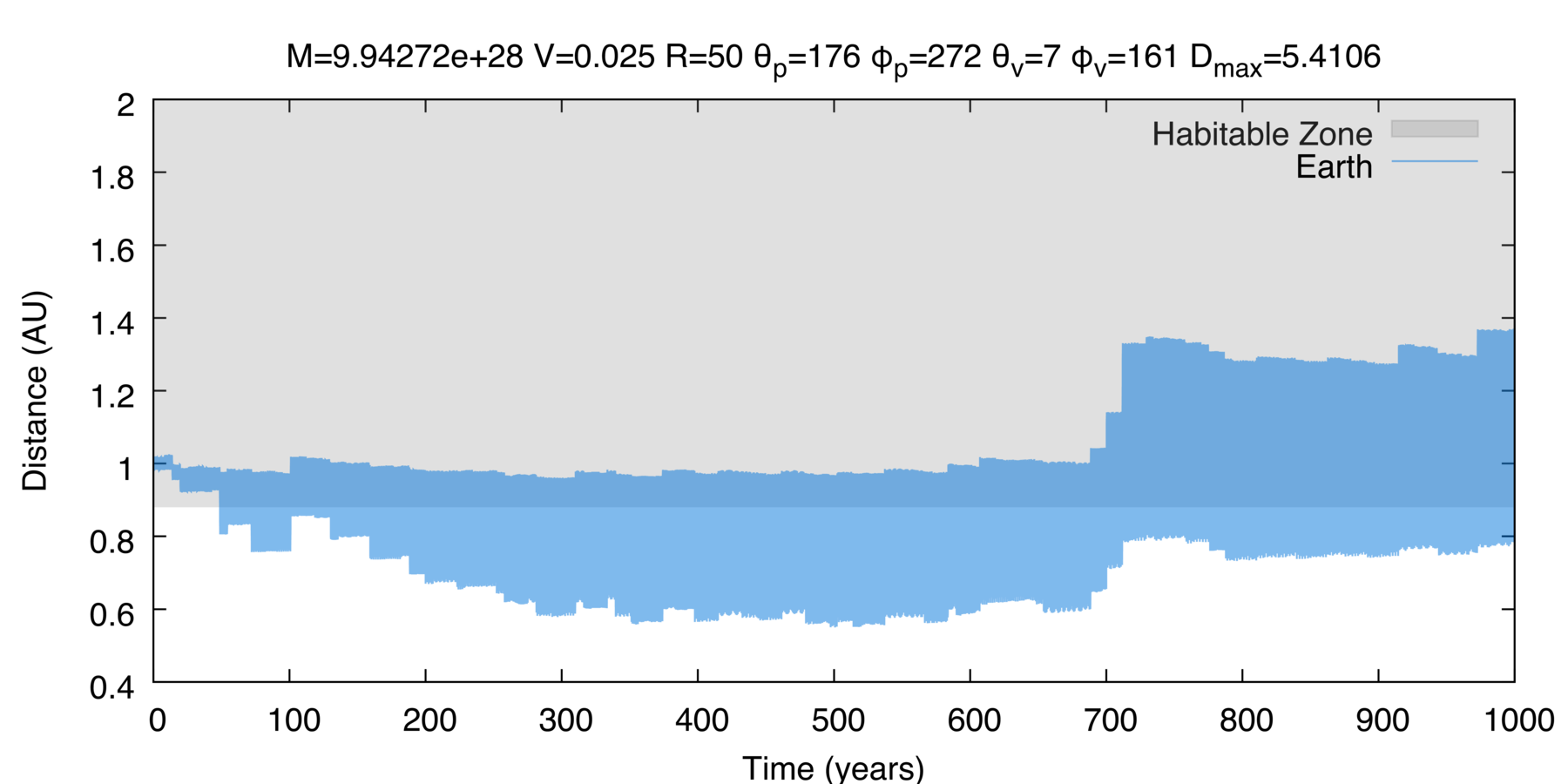
Black Dwarf: Effects on Earth's Orbit

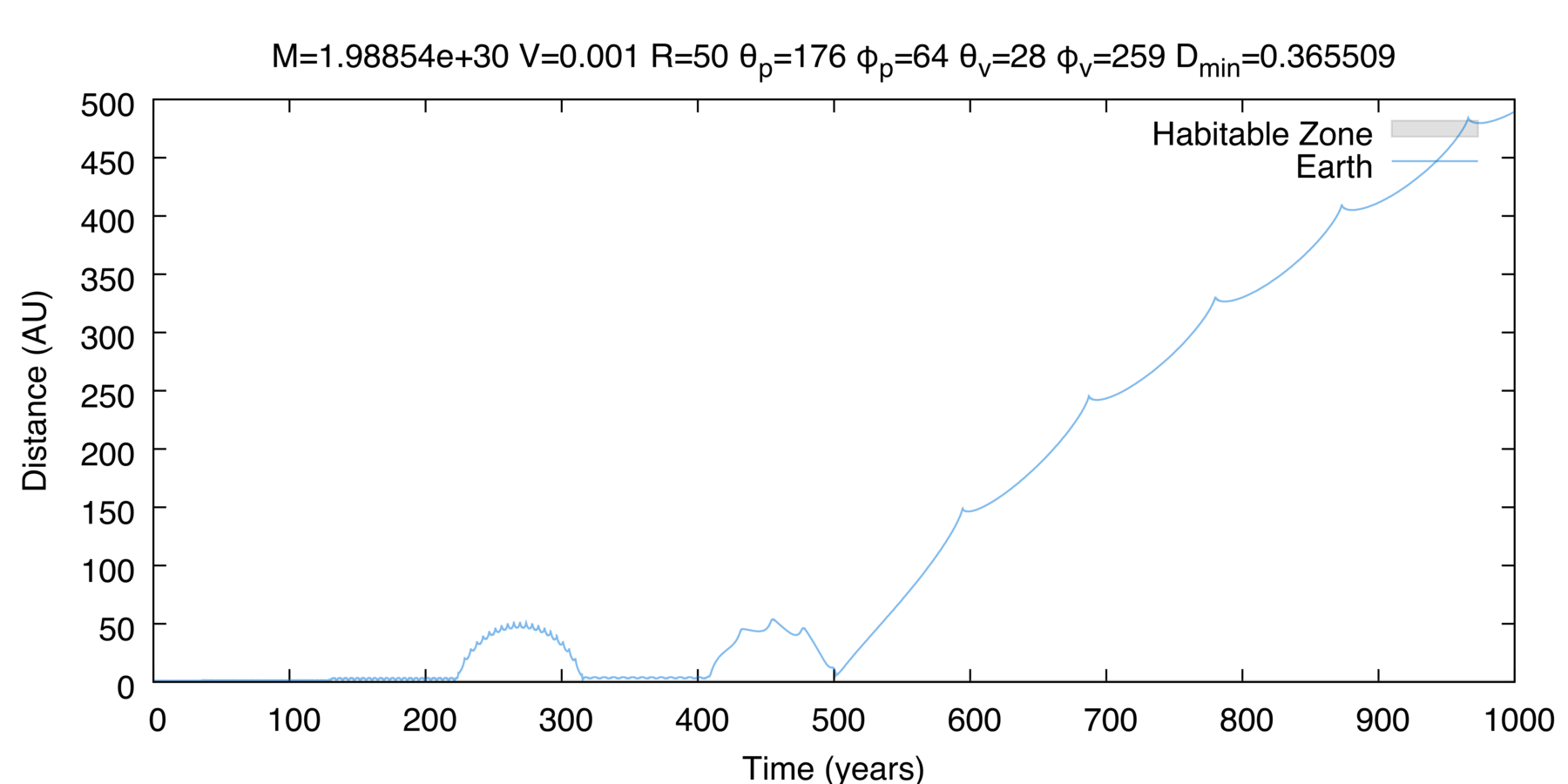
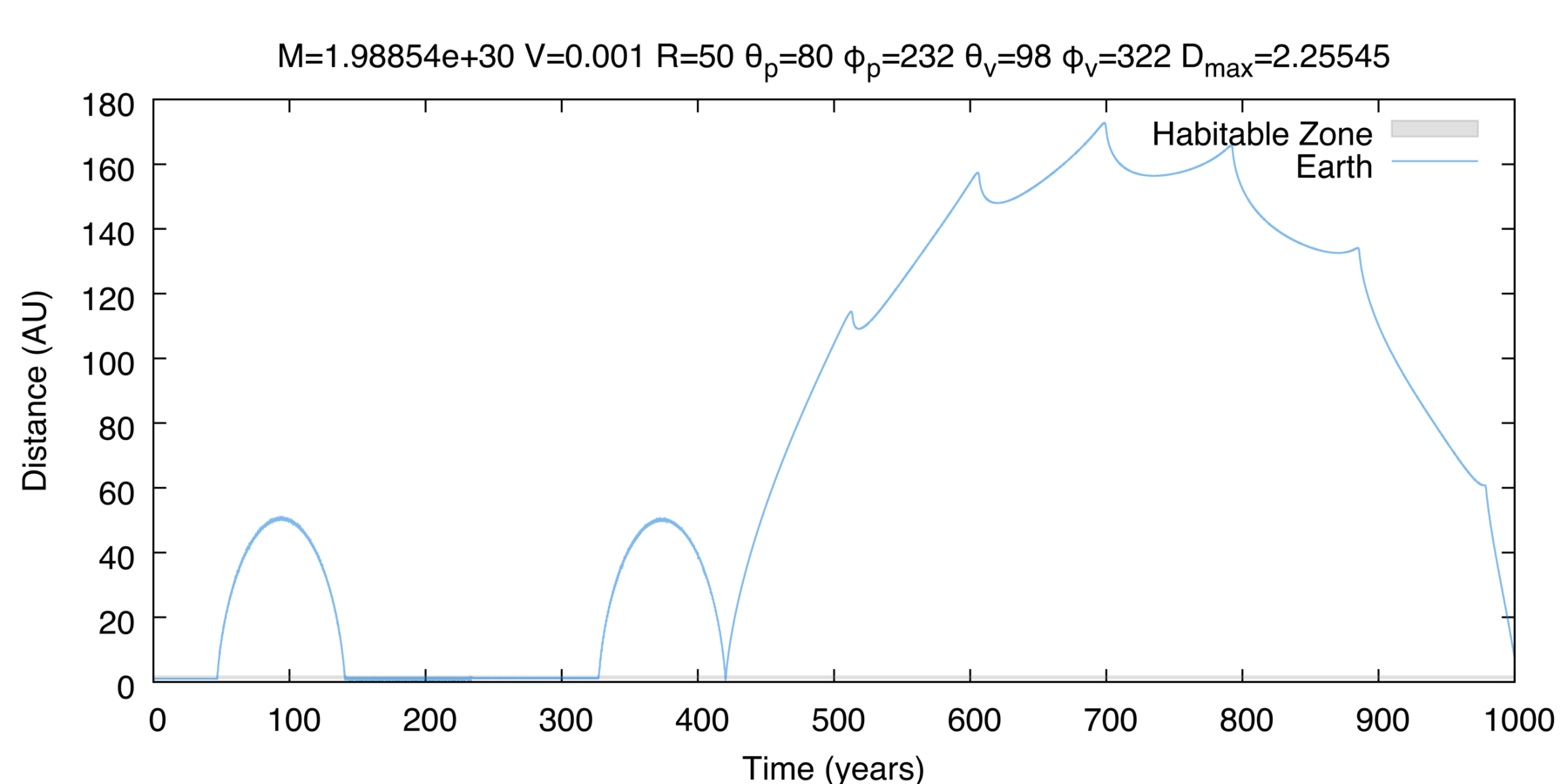
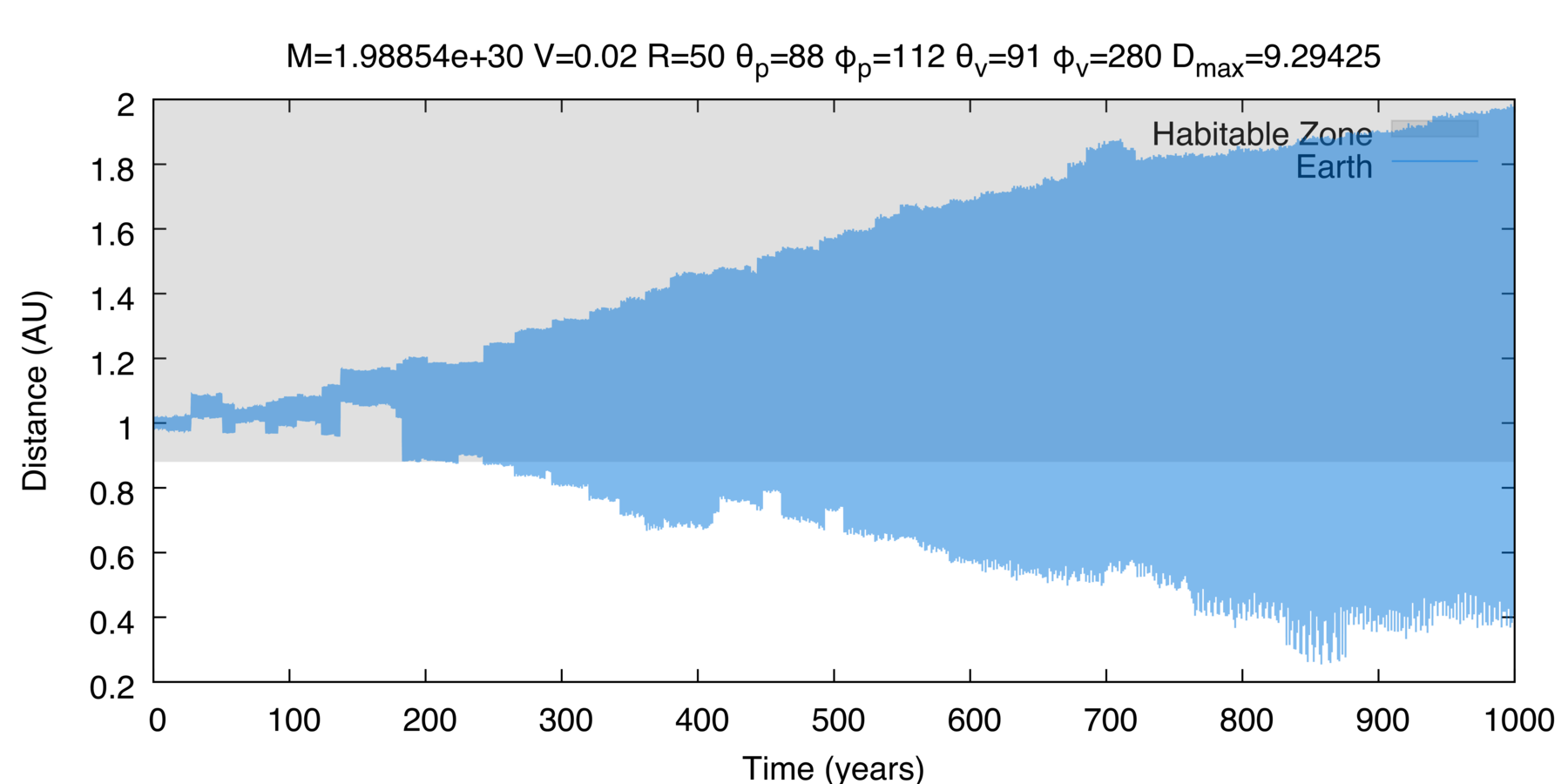
Black Hole: Effects on Earth's Orbit
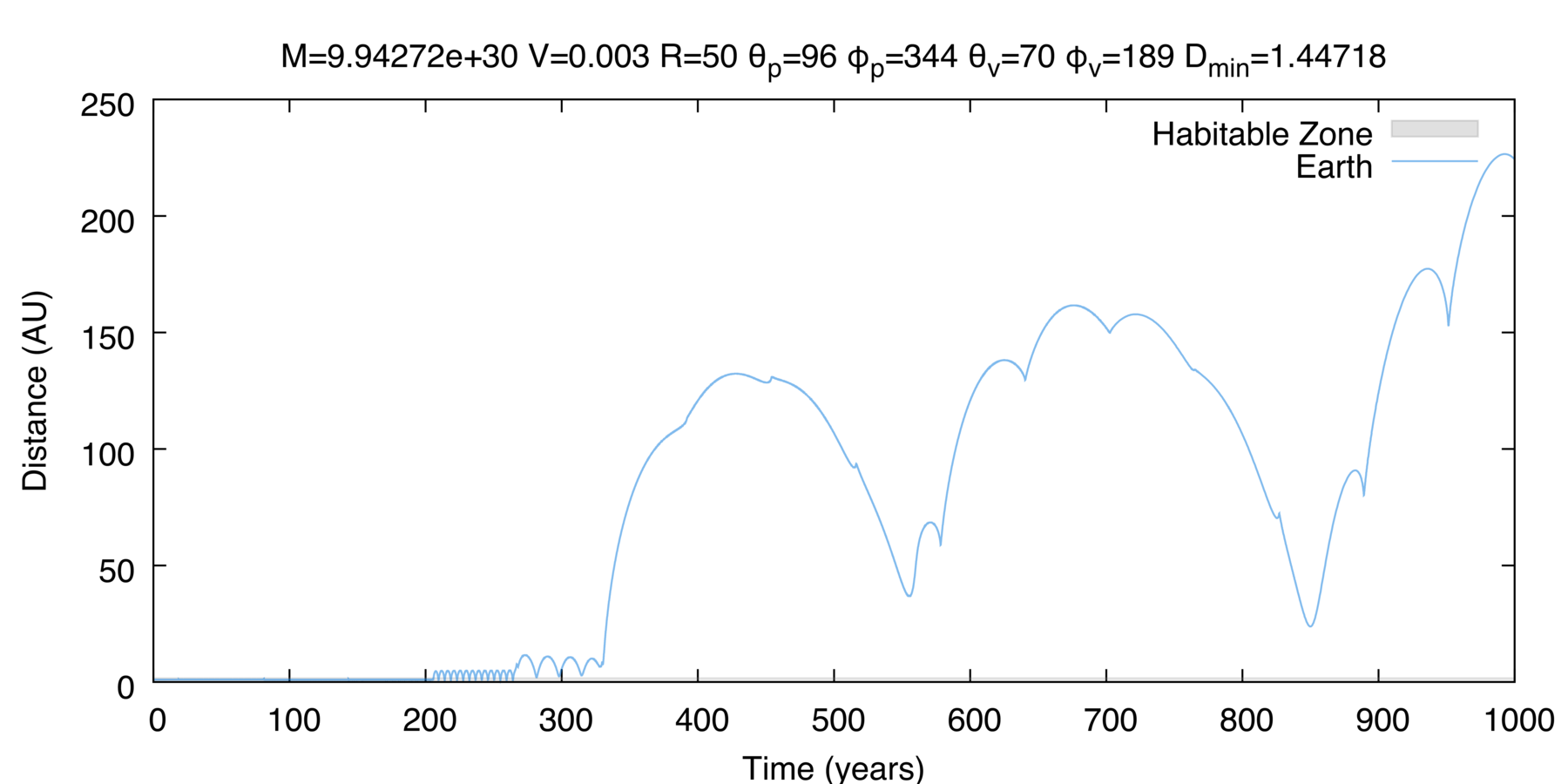
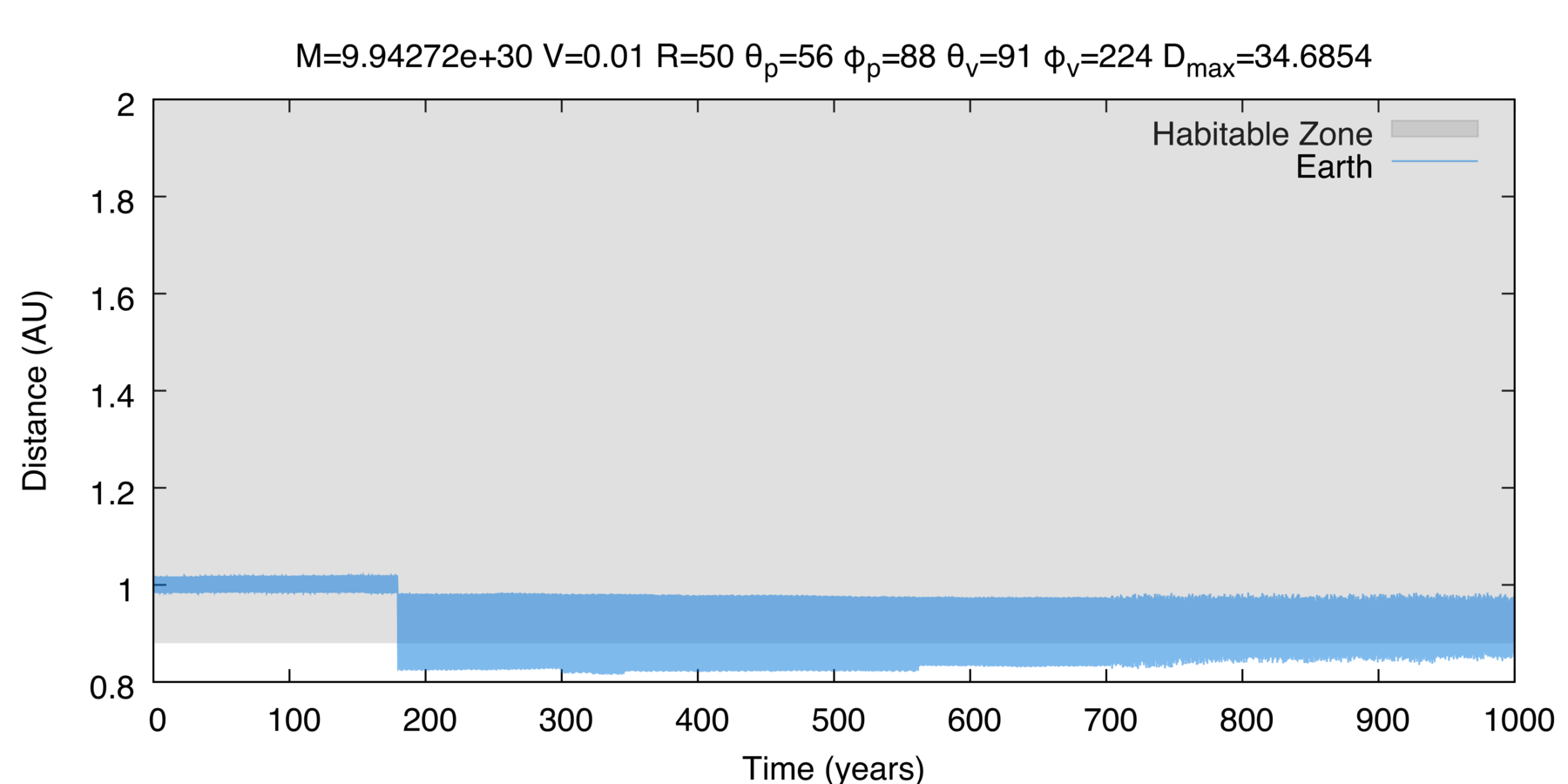
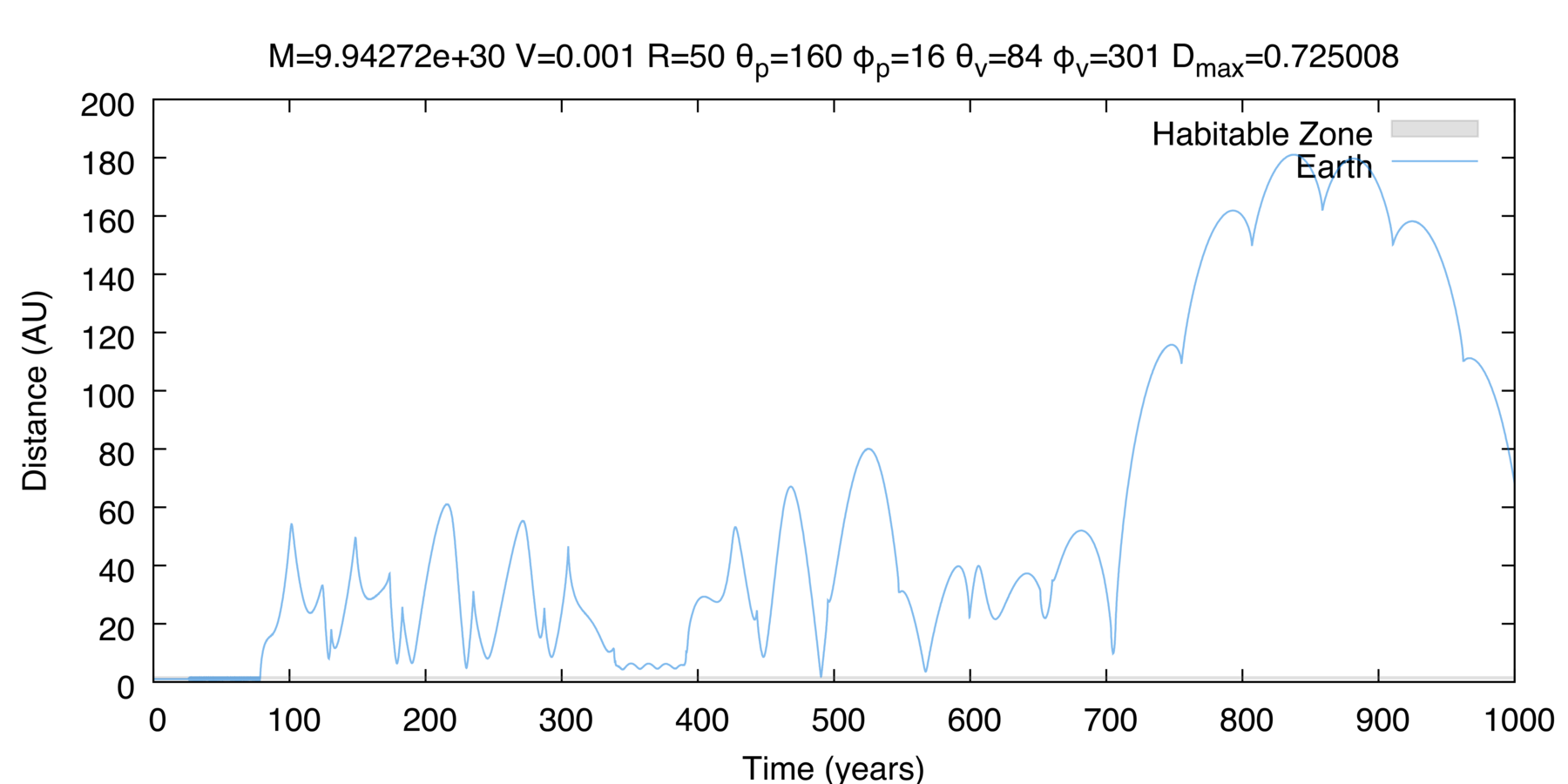
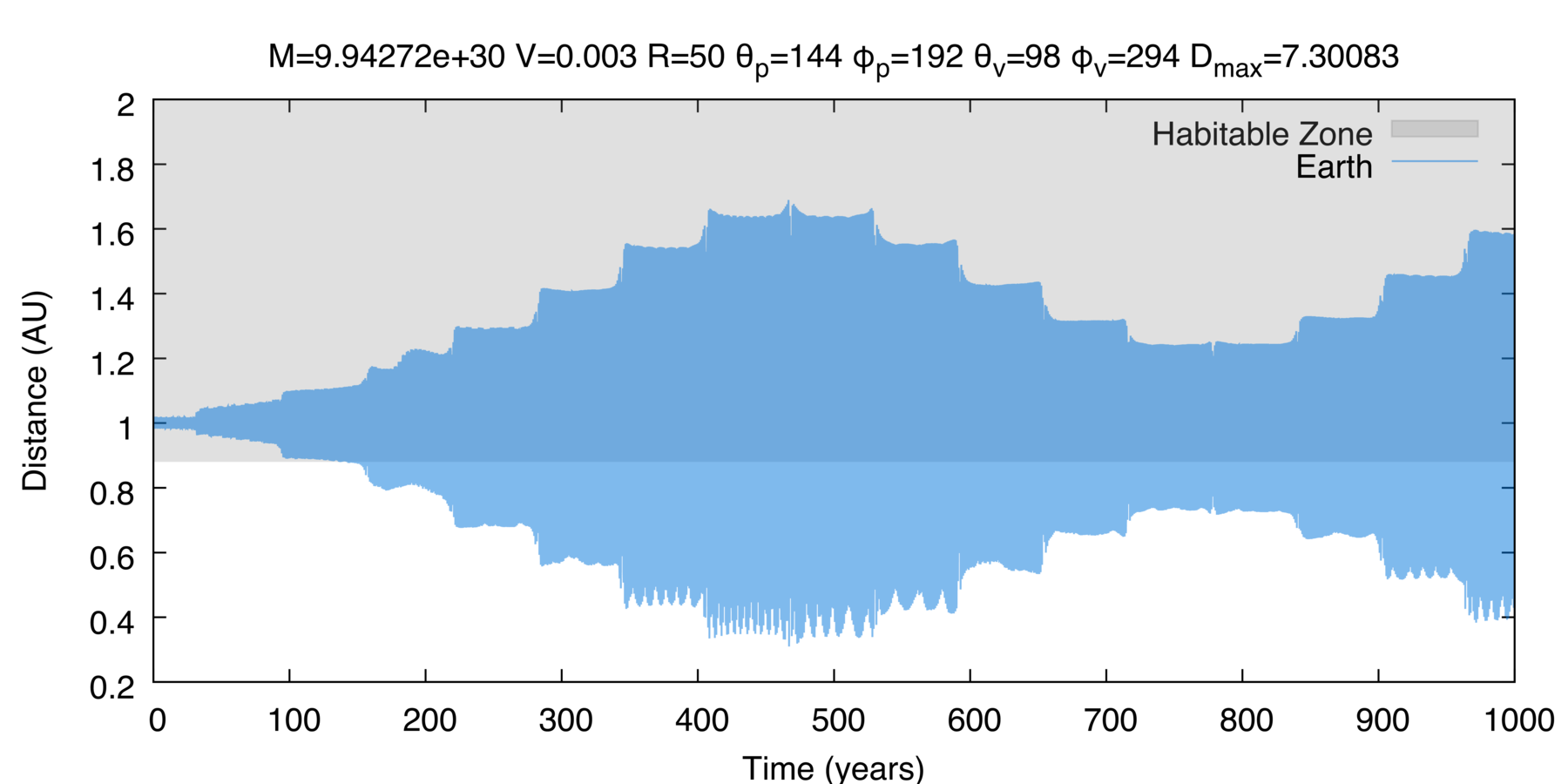
Conclusions
- Probability for life extinction depends on the mass and velocity of the incoming celestial body.
- Brown star can get as close as Jupiter's orbit before knocking the Earth out of the habitable zone.
- Stellar black hole can get closer than Earth's orbit to the Sun without knocking the Earth out of the habitable zone.
- Future steps include finding the average amount of time the Earth has before all life is killed.
References
- European Southern Observatory. Hertzsprung-Russell Diagram. https://www.eso.org/public/images/eso0728c/
- European Space Agency. Location of comets in the Solar System. http://sci.esa.int/jump.cfm?oid=49390
- Rein, Hanno, and S-F. Liu. "REBOUND: an open-source multi-purpose N-body code for collisional dynamics." Astronomy & Astrophysics 537 (2012): A128.
- Wikipedia. February 1 2018. Dark star (Newtonian mechanics). https://en.wikipedia.org/wiki/Dark_star_(Newtonian_mechanics%29
- Dehnen, Walter, and Justin I. Read. "N-body simulations of gravitational dynamics." The European Physical Journal Plus 126.5 (2011): 1-28.
- Wikipedia. February 17 2018. Brown dwarf. https://en.wikipedia.org/wiki/Brown_dwarf
- "JPL Solar System Dynamics." NASA, NASA, http://ssd.jpl.nasa.gov/.
- Wikipedia. January 4 2018. Luhman 16. https://en.wikipedia.org/wiki/Luhman_16
- Wikipedia. February 10 2018. Solar System. https://en.wikipedia.org/wiki/Solar_System
- NASA. JPL Horizons System. JPL. https://ssd.jpl.nasa.gov/horizons.cgi
- Burgasser, Adam J. "Brown dwarfs: Failed stars, super Jupiters." Physics Today 61.6 (2008): 70.
- Mamajek, Eric E., et al. "The Closest Known Flyby of a Star to the Solar System." The Astrophysical Journal Letters 800.1 (2015): L17.
- Binney, James, and Scott Tremaine. Galactic dynamics. Princeton university press, 2011.